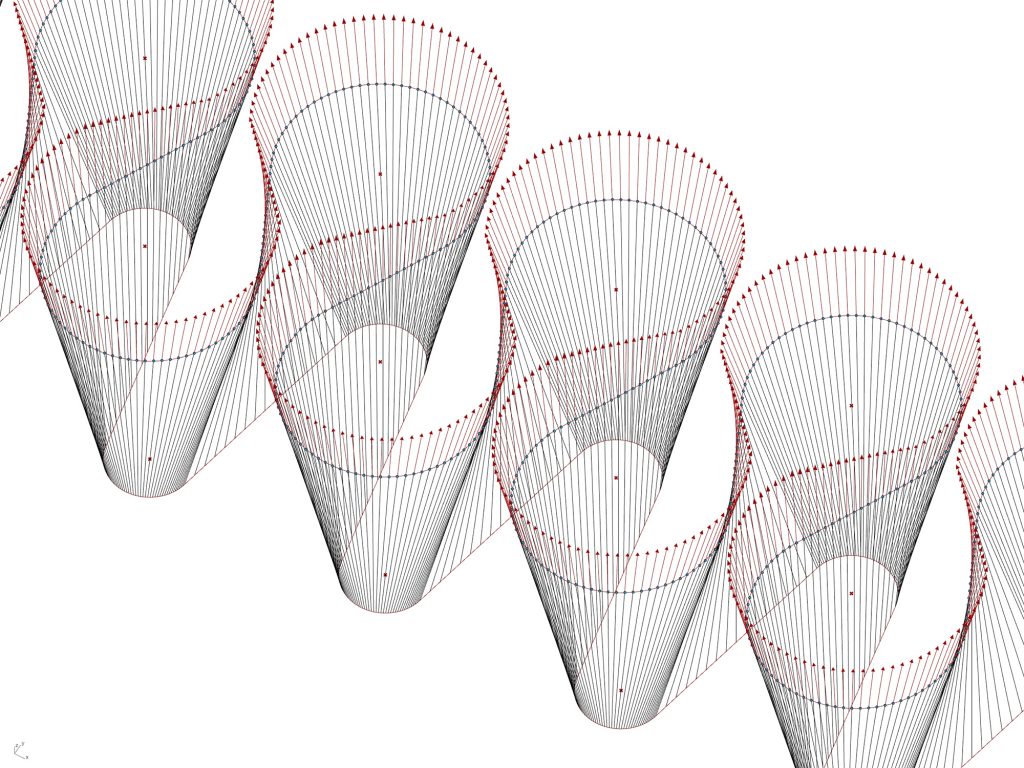
This research explores the topological and structural properties of disclinations applied to elastic sheets and suggests their potential at an architectural scale. The basic topological concept of a disclination acknowledges the role of the “defect” in the composition of a material structure. However, the geometric principles of disclinations can be applied to material systems at multiple scales. Thin sheets absorb the strain induced by disclinations by buckling into repeatable, complex forms with inherently rationalized curvature. For example, wedge disclinations in flexible sheets result in surfaces that do not reveal the topological defect that creates curvature through self-reaction; a rather elegant formal and structural condition.
Work thus far has been based on the geometric principles of various disclination types and proposes a variety of physical and computational form-finding techniques that demonstrate these principles in an architectural workflow. Several large-scale prototypes of architectural disclinations are proposed as a contribution to research on bending-active plate structures and their potential application in moldless composite construction techniques.
Nick Bruscia, with students in the Fall 2019 and Spring 2021 STRG sponsored research studios.
Fall 2019: Heidi Flores, Rossella Giangreco-Marotta, James Hedger, Russ Kolker, Rania Moussa, Rebecca Flanagan, Stanicka Mathurin, Mehrad Naghizadeh, Kajal Patel, Yogesh Ravichandar, Tim Zeng.
Spring 2021: Josh Barzideh, Tyler Beerse, Tom Cleary, Camilo Copete, Bhalendu Gautam, Samantha Goembel, Marissa Hayden, Ollie He, Nick Hills, Jamie Jones, Lovepreet Kaur, Ben Starr.
Supported by a grant from the Nohmura Foundation for Membrane Structures Technology.